3.19 演習 〜 微分
問 3.55 (微分係数)
点 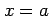 における関数
における関数 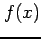 の微分係数
の微分係数 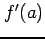 の定義を述べよ.
の定義を述べよ.
問 3.56 (微分可能)
次の関数について(i) 関数 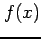 のグラフを描け.
(ii)
のグラフを描け.
(ii) 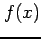 の微分不可能な点を述べよ.
また,この点における右微分係数,左微分係数を求めよ.
(iii) 微分可能な範囲で導関数
の微分不可能な点を述べよ.
また,この点における右微分係数,左微分係数を求めよ.
(iii) 微分可能な範囲で導関数 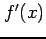 を求めよ.
(iv)
を求めよ.
(iv) 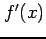 のグラフを描け.
のグラフを描け.
(1)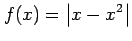 (2)
(2)
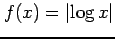
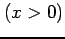 (3)
(3)
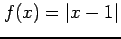
(1)
問 3.57 (初等関数の導関数)
次の関数の導関数を書け.
(1)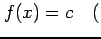 $c$:定数
$c$:定数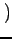 (2)
(2)
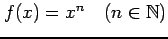 (3)
(3)
![$ f(x)=\sqrt[n]{x}\quad(n\in\mathbb{N})$](img1164.png)
(4)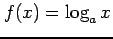 (5)
(5)
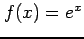 (6)
(6)
 (7)
(7)
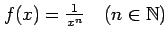
(8)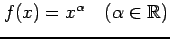 (9)
(9)
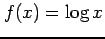 (10)
(10)
 (11)
(11)
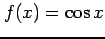
(12) (13)
(13)
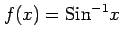 (14)
(14)
 (15)
(15)

(16)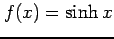 (17)
(17)
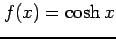 (18)
(18)
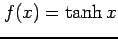 (19)
(19)

(20)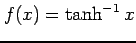 (21)
(21)
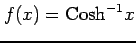
(1)
(4)
(8)
(12)
(16)
(20)
問 3.58 (導関数の導出)
次の関数の導関数を導出せよ.
(1)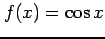 (2)
(2)
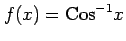 (3)
(3)
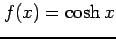 (4)
(4)
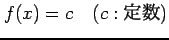
(5)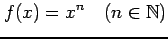 (6)
(6)
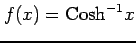 (7)
(7)
 (8)
(8)
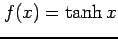
(9)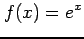 ただし,
ただし,
 が導出させているとして議論せよ.
が導出させているとして議論せよ.
(1)
(5)
(9)
問 3.59 (導関数の導出)

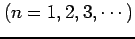 が成り立つことを示せ.
が成り立つことを示せ.

問 3.60 (導関数の導出)
次を示せ.
(1) (2)
(2)
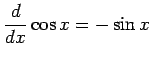 (3)
(3)
 (4)
(4)
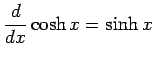
(1)
 (2)
(2)
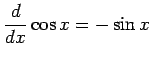 (3)
(3)
 (4)
(4)
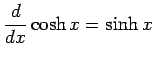
問 3.61 (微分の性質)
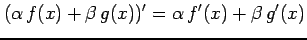 を証明せよ.
を証明せよ.
問 3.62 (微分の計算)
次の関数の導関数を求めよ.
(1)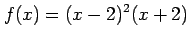 (2)
(2)
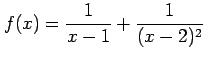 (3)
(3)
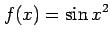
(4)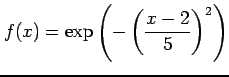 (5)
(5)
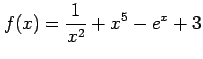 (6)
(6)
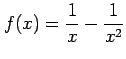
(7)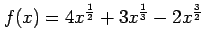 (8)
(8)
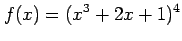 (9)
(9)
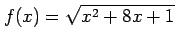
(10)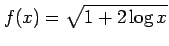 (11)
(11)
![$ \displaystyle{f(x)=\sqrt[\leftroot{2} \uproot{2} 3]{\frac{x+2}{x^2+1}}}$](img1208.png) (12)
(12)
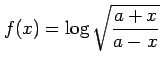
(13)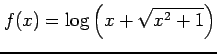 (14)
(14)

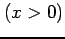 (15)
(15)
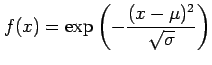
(16)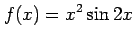 (17)
(17)
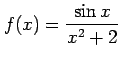 (18)
(18)
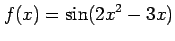 (19)
(19)
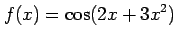
(20) (21)
(21)
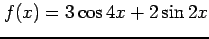 (22)
(22)

(23)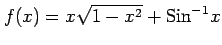 (24)
(24)
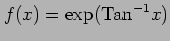 (25)
(25)
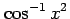 (26)
(26)
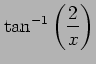
(27)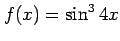 (28)
(28)
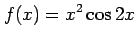 (29)
(29)
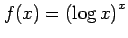
(30)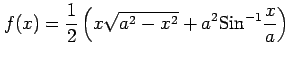
(1)
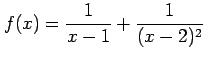 (3)
(3)
(4)
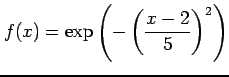 (5)
(5)
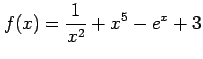 (6)
(6)
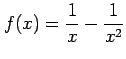
(7)
(10)
![$ \displaystyle{f(x)=\sqrt[\leftroot{2} \uproot{2} 3]{\frac{x+2}{x^2+1}}}$](img1208.png) (12)
(12)
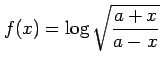
(13)
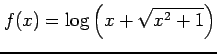 (14)
(14)
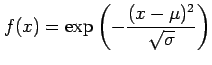
(16)
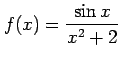 (18)
(18)
(20)
(23)
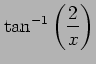
(27)
(30)
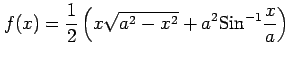
問 3.63 (高階導関数)
次の関数の高階導関数を求めよ.
(1)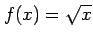 (2)
(2)
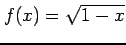 (3)
(3)
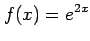 (4)
(4)
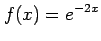 (5)
(5)
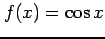
(6)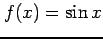 (7)
(7)
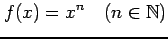 (8)
(8)

(1)
(6)
問 3.64 (高階導関数)
関数
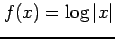 について
について 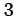 階までの
導関数を求め,それぞれのグラフを描け.
階までの
導関数を求め,それぞれのグラフを描け.
問 3.65 (接線)
次の関数について
(i) 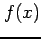 のグラフを書け.
(ii)
のグラフを書け.
(ii) 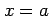 における接線の方程式を求めよ.
(iii) 接線のグラフを書け.
における接線の方程式を求めよ.
(iii) 接線のグラフを書け.
(1) ,
, 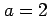 ,
(2)
,
(2)
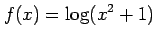 ,
, 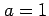 ,
(3)
,
(3)
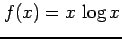 ,
, 
(4) ,
,
 (5)
(5)
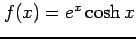 ,
, 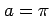
(6)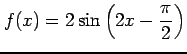 ,
,
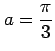
(1)
(4)
 ,
,
 (5)
(5)
(6)
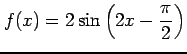 ,
,
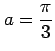
問 3.66 (接線)
関数
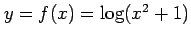 について
(i) 点
について
(i) 点 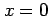 ,
,
 における接線
における接線 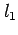 ,
, 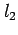 の方程式を書け.
(ii)
の方程式を書け.
(ii) 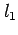 と
と 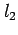 の交点を求めよ.
(iii) 関数
の交点を求めよ.
(iii) 関数 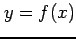 , 接線
, 接線 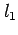 ,
, 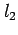 のグラフを同一のグラフ上に描け.
のグラフを同一のグラフ上に描け.
問 3.67 (なめらかさ)
次の関数が 級の関数であるか不連続関数であるか答えよ.
級の関数であるか不連続関数であるか答えよ.
(1) (2)
(2)
 (3)
(3)
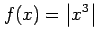 (4)
(4)

(5)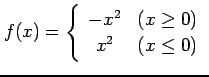 (6)
(6)
 (7)
(7)
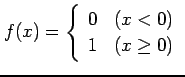
(8)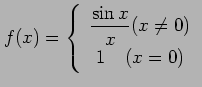
(1)
(5)
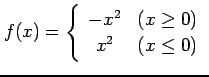 (6)
(6)
 (7)
(7)
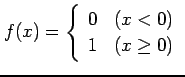
(8)
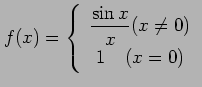
平成19年10月3日