Next: ちょっとまとめ Up: 微分法 Previous: 逆双曲線関数の微分 Contents
高階導関数
定義 3.35 (高階導関数)
関数  が微分可能のとき,
が微分可能のとき, の導関数
の導関数
を2 階導関数(second order derivative)という. このとき は
2 回微分可能(two times differentiable)と呼ぶ.
同様に
は
2 回微分可能(two times differentiable)と呼ぶ.
同様に  を
を  回繰り返し微分した関数を
回繰り返し微分した関数を
 階導関数(
階導関数( -th order derivative)といい,
-th order derivative)といい,
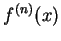 と書き表わす.
関数
と書き表わす.
関数
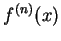 は
は
と再帰的に定義する. ただし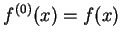 とする.
とする.
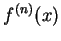 が存在するとき
が存在するとき  は
は
 回微分可能(
回微分可能( times differentiable)という.
times differentiable)という.
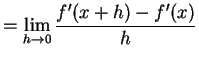 |
(380) |
を2 階導関数(second order derivative)という. このとき
 |
(381) |
と再帰的に定義する. ただし
例 3.36 (高階導関数の計算例)
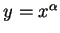 の高階導関数を求める.
の高階導関数を求める.
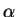 が自然数ではないとき,
が自然数ではないとき,
を得る.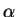 が自然数
が自然数  のとき,
のとき,
を得る.
| (382) | ||
| (383) | ||
| (384) | ||
| (385) | ||
| (386) | ||
を得る.
| (387) | ||
| (388) | ||
| (389) | ||
| (390) | ||
| (391) | ||
| (392) | ||
| (393) | ||
| (394) | ||
を得る.
例 3.37 (高階導関数の計算例)
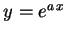 の高階導関数を求める.
合成関数の微分を繰り返して
の高階導関数を求める.
合成関数の微分を繰り返して
を得る.
| (395) | ||
| (396) | ||
| (397) | ||
| (398) | ||
| (399) |
を得る.
例 3.38 (高階導関数の計算例)
ただし
と定義する.
| (400) | ||
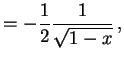 |
(401) | |
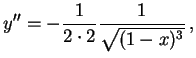 |
(402) | |
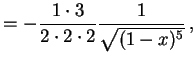 |
(403) | |
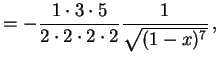 |
(404) | |
| (405) | ||
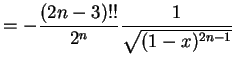 |
(406) |
ただし
| (407) | ||
| (408) | ||
| (409) |
と定義する.
問 3.39
教科書(p.52)問題 3-3.
Next: ちょっとまとめ Up: 微分法 Previous: 逆双曲線関数の微分 Contents
Kondo Koichi
Created at 2002/09/12