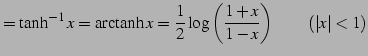Next: 14 関数の極限 Up: 2 関数 Previous: 12 双曲線関数 Contents
13 逆双曲線関数
双曲線関数の逆関数は 逆双曲線関数(inverse hyperbolic function) と呼び,
と表される. 読み方は上から hyperbolic arc sine, hyperbolic arc cosine, hyperbolic arc tangent である.
問 2.46 (逆双曲線関数のグラフ)
逆双曲線関数の概形を書け.
問 2.47 (逆双曲線関数の対数関数表示)
逆双曲線関数が(![[*]](./icons/crossref.png) )-(
)-(![[*]](./icons/crossref.png) )のように
対数関数を用いて書き表されることを示せ.
)のように
対数関数を用いて書き表されることを示せ.
を得る.
(答え)
![]() とおく.
逆に書けば
とおく.
逆に書けば
![]() である.
これより
である.
これより
| (239) | ||
| (240) | ||
| (241) | ||
| (242) | ||
| (243) | ||
| (244) | ||
| この条件のもとでは複合の`` |
(245) | |
| (246) | ||
| (247) |
を得る.
Next: 14 関数の極限 Up: 2 関数 Previous: 12 双曲線関数 Contents
Kondo Koichi
Created at 2003/08/29