1.8 合成写像
定義 1.30 (合成写像)
写像 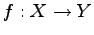 ,
, 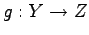 に対して,
に対して,
で定義される写像を
と表記し,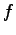 と
と 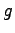 の合成写像(composite)という.
の合成写像(composite)という.
で定義される写像を
と表記し,
例 1.31 (合成写像の具体例)
写像
の合成写像は
である.
の合成写像は
である.
定理 1.32 (合成写像の性質)
写像の合成に対して結合則
が成り立つ.
が成り立つ.
平成20年2月2日