2.32 調和関数
定義 2.136 (ラプラス演算子)
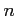 次元座標
次元座標
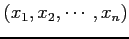 の空間の
ラプラス演算子(Laplace operator)または
ラプラシアン(Laplacian)は
の空間の
ラプラス演算子(Laplace operator)または
ラプラシアン(Laplacian)は
により定義される.
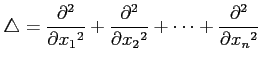 |
により定義される.
定義 2.137 (調和関数)
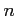 変数関数
変数関数
 が
が
をみたすとき, を調和関数(harmonic function)という.
を調和関数(harmonic function)という.
をみたすとき,
例 2.138 (調和関数)
次の関数は調和関数である.
 |
||
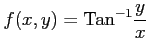 |
||
平成21年12月2日