5.21 演習問題 〜 行列の対角化
問 5.57 (対角化)
次の行列  を対角化せよ.また
を対角化せよ.また  を求めよ.
を求めよ.
(1)
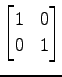 (2)
(2)
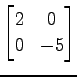 (3)
(3)
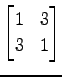 (4)
(4)
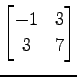 (5)
(5)
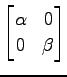 (6)
(6)
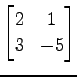
(7)
 (8)
(8)
 (9)
(9)
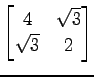 (10)
(10)
 (11)
(11)
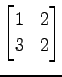
(12)
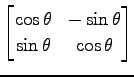 (13)
(13)
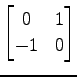 (14)
(14)
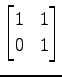 (15)
(15)
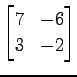 (16)
(16)
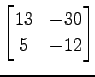
(17)
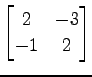 (18)
(18)
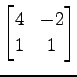 (19)
(19)
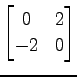 (20)
(20)
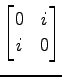 (21)
(21)
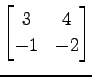
(22)
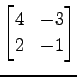 (23)
(23)
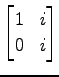 (24)
(24)
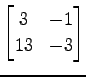 (25)
(25)
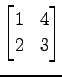 (26)
(26)
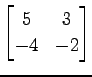
(27)
 (28)
(28)
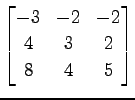 (29)
(29)
 (30)
(30)

(31)
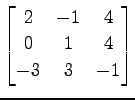 (32)
(32)
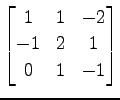 (33)
(33)
 (34)
(34)

(35)
 (36)
(36)
 (37)
(37)
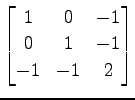 (38)
(38)

(39)
 (40)
(40)
 (41)
(41)
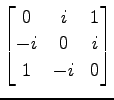 (42)
(42)
 (43)
(43)
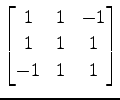
(44)
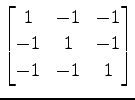 (45)
(45)
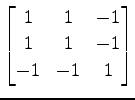 (46)
(46)
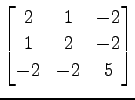 (47)
(47)

(48)
 (49)
(49)
 (50)
(50)
 (51)
(51)

(52)
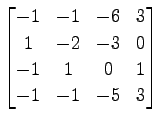 (53)
(53)

平成20年2月2日