3.9 演習問題 〜 部分空間
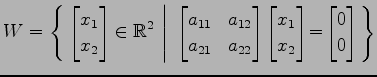
問 3.40 (部分空間)
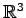 の部分集合
の部分集合 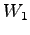 ,
, 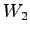 が
が
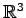 の部分空間であるかどうか示せ.
の部分空間であるかどうか示せ.
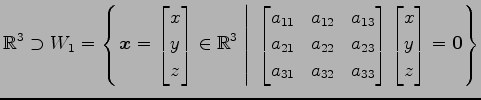
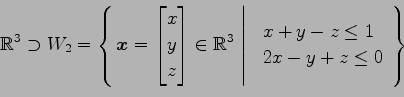
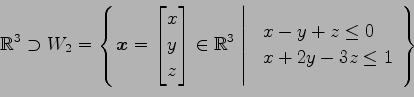
(1)
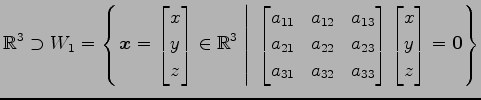
(2)
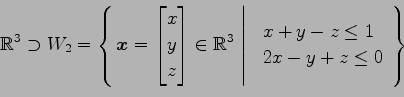
(3)
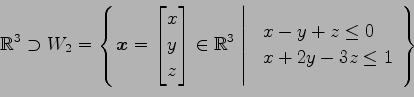
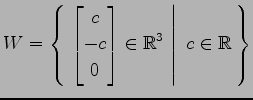
問 3.41 (部分空間)
次の
 の部分集合
の部分集合  ,
, 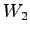 ,
,  ,
, 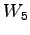 のうち
部分空間となるものはどれか.
のうち
部分空間となるものはどれか.
(1)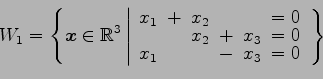
(2)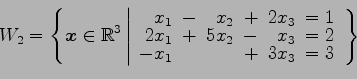
(3)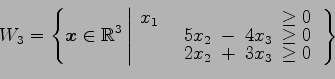
(4)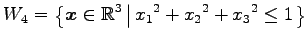
(5)![$ \displaystyle{
W_{5}=
\left\{
\vec{x}\in\mathbb{R}^3
\left\vert\,
\left(
\begi...
...in{bmatrix}
1 \\ [-1ex]
1 \\ [-1ex]
1
\end{bmatrix}\right)=0
\right.
\right\}
}$](img604.png)
(1)
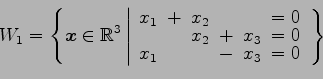
(2)
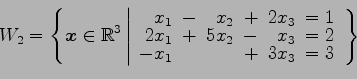
(3)
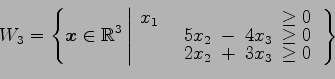
(4)
(5)
![$ \displaystyle{
W_{5}=
\left\{
\vec{x}\in\mathbb{R}^3
\left\vert\,
\left(
\begi...
...in{bmatrix}
1 \\ [-1ex]
1 \\ [-1ex]
1
\end{bmatrix}\right)=0
\right.
\right\}
}$](img604.png)
問 3.42 (部分空間)
ベクトル空間
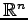 において,
部分集合
において,
部分集合  は部分空間であるか述べよ.
は部分空間であるか述べよ.
(1)
![]() (2)
(2)
![]() (ただし,
(ただし,
![]() )
)
(3)
![]() (4)
(4)
![]()
(5)
![]() (
(
![]() はあるベクトル)
はあるベクトル)
(6)
![]()
問 3.43 (部分空間)
ベクトル空間
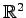 において,
部分集合
において,
部分集合  は部分空間であるか述べよ.
は部分空間であるか述べよ.
(1)
 (2)
(2)

(3)
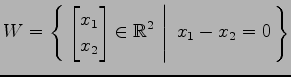 (4)
(4)

(5)
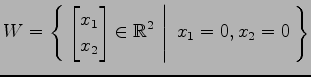 (6)
(6)
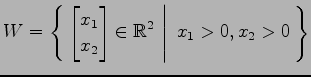
(7)
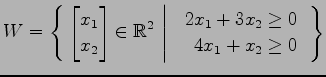 (8)
(8)

(9)
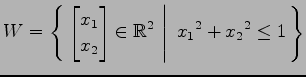 (10)
(10)
(11)
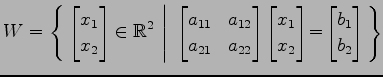
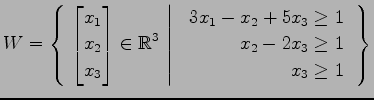
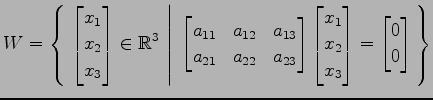
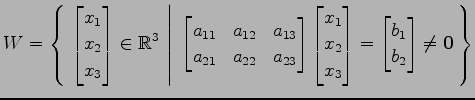
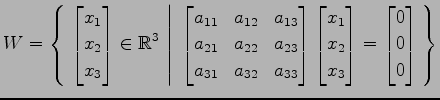
問 3.44 (部分空間)
ベクトル空間
 において,
部分集合
において,
部分集合  は部分空間であるか述べよ.
は部分空間であるか述べよ.
(1)
 (2)
(2)
(3)
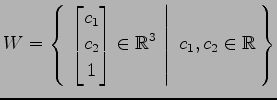 (4)
(4)
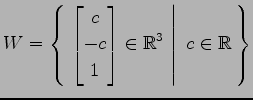
(5)
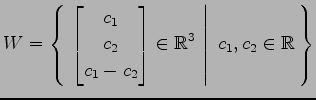 (6)
(6)
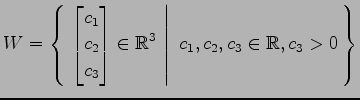 (7)
(7)
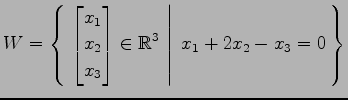 (8)
(8)
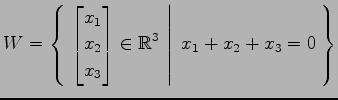
(9)
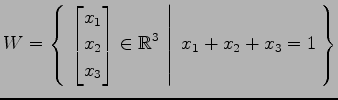 (10)
(10)
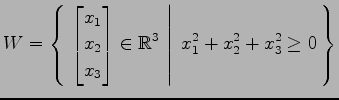 (11)
(11)
 (12)
(12)
(13)
(14)
(15)
(16)
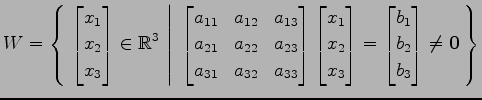
問 3.45 (部分空間)
ベクトル空間
![$ \mathbb{R}[x]_3$](img561.png) において,
部分集合
において,
部分集合  は部分空間であるか述べよ.
は部分空間であるか述べよ.
(1)
![]() (2)
(2)
![]()
(3)
![]() (4)
(4)
![]() (5)
(5)
![]() (6)
(6)
![]() (7)
(7)
![]() (8)
(8)
![]()
(9)
![]() (10)
(10)
![]()
(11)
![]() (12)
(12)
![]()
(13)
![]()
(14)
![]()
(15)
![]()
(16)
![]()
(17)
![]()
(18)
![]()
平成20年2月2日