3.41 演習問題 〜 正規直交基底
問 3.170 (正規直交基底)
 の正規直交基底は
の正規直交基底は
のみであることを示せ.
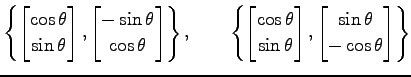 |
のみであることを示せ.
問 3.171 (正規直交基底)
次のベクトルの組が
 の正規直交基底であることを示せ.
の正規直交基底であることを示せ.
 |
問 3.172 (グラム・シュミットの直交化法)
次の基底を正規直交化せよ.
(1)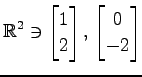 (2)
(2)
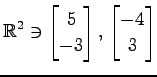 (3)
(3)
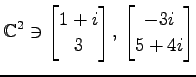
(4)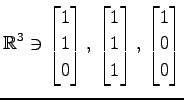 (5)
(5)
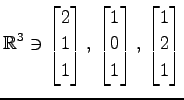 (6)
(6)
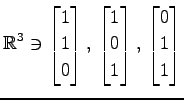
(7)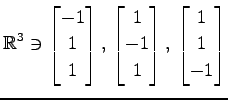 (8)
(8)
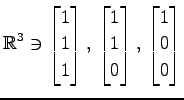 (9)
(9)
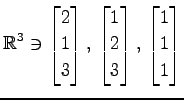
(10)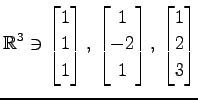 (11)
(11)
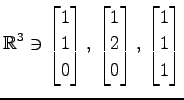 (12)
(12)
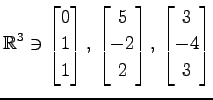
(13)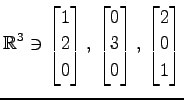 (14)
(14)
 (15)
(15)
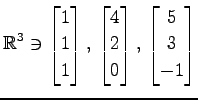
(16)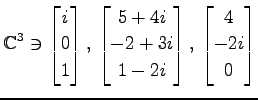 (17)
(17)
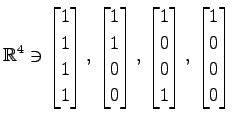
(18)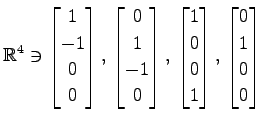 (19)
(19)
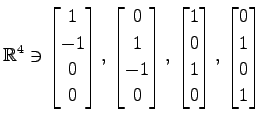
(20)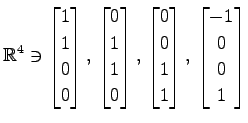 (21)
(21)

(22)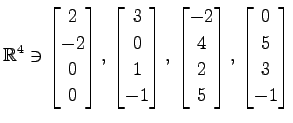 (23)
(23)

(1)
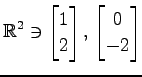 (2)
(2)
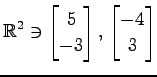 (3)
(3)
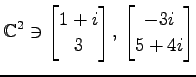
(4)
 (5)
(5)
 (6)
(6)

(7)
 (8)
(8)
 (9)
(9)

(10)
 (11)
(11)
 (12)
(12)

(13)
 (14)
(14)
 (15)
(15)

(16)
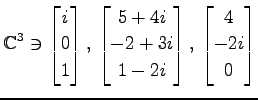 (17)
(17)

(18)
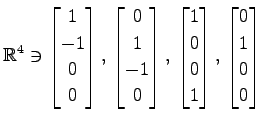 (19)
(19)
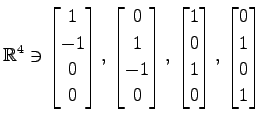
(20)
 (21)
(21)

(22)
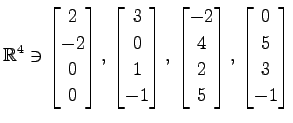 (23)
(23)

問 3.173 (正規直交基底における座標)
前問で得た正規直交基底における次のベクトルの座標を求めよ.
(1)-(2)
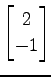 (3)
(3)
 (4)-(15)
(4)-(15)
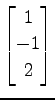 (16)
(16)
 (17)-(22)
(17)-(22)
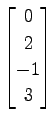 (23)
(23)
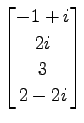
問 3.174 (グラム・シュミットの直交化法)
 において
において
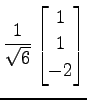 を含む正規直交基底を2 組つくれ.
を含む正規直交基底を2 組つくれ.
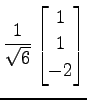 を含む正規直交基底を2 組つくれ.
を含む正規直交基底を2 組つくれ.
問 3.175 (グラム・シュミットの直交化法)
![$ \mathbb{R}[x]_2$](img412.png) の基底
(1)
の基底
(1)
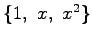 (2)
(2)
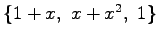 を正規直交化せよ.
ただし,内積は
を正規直交化せよ.
ただし,内積は
とする.
 |
とする.
問 3.176 (グラム・シュミットの直交化法)
![$ \mathbb{R}[x]_n$](img375.png) の基底
の基底 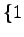 ,
, 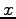 ,
, 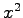 ,
, 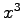 ,
, 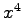 ,
,  ,
, 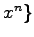 を
正規直交化せよ.
ただし,内積は前問と同じとする.
を
正規直交化せよ.
ただし,内積は前問と同じとする.
平成20年2月2日